Maurits Cornelis Escher: The Mathematical Vision of a Graphic Artist

Maurits Cornelis Escher was an extraordinary graphic artist who left us his work as an example of an exceptional manipulation of space, time, and perspective. Through his extensive search for the regular division of the plane, he made countless engravings on wood and limestone where he experimented with the repetition, reflection, rotation, and multiplication of geometric shapes (squares, rectangles, triangles, hexagons, etc.) which, by constantly studying and transforming them, gave rise to forms where reptiles, birds, fish, goblins, demons, angels and angels emerge. An infinite number of beings that, when captured on paper, acquire movement and life on the plane; fantastic forms through which the artist expresses his ideas, each one with its history.

The theme in many of his prints revolves around the illusion of space, the representation of two-dimensionality, and three-dimensionality on a flat surface. His drawings manage to capture this illusion of volume. His work is the result of a reasoned construction that we perceive as a spatial drawing, with volume within the plane. There are several examples where he shows us, through his engravings, impossible compositions, although their context is rational.

Crystals were also of great interest to this artist. The innumerable possibilities offered by crystals to compose figures in space and the shadows they produce caused great admiration and inspiration to Escher.

Maurits Cornelis Escher Chronological Biography

1898. M. C. Escher was born on June 17 in Leeuwarden, in the Dutch province of Friesland, in the north of Holland. At the age of five, he moved with his family to Arnhem. From an early age, he showed an interest in drawing, mathematics, and astronomy. He studied at the middle school in Arnhem where he received drawing lessons from F.W. van der Haagen, whose linoleum engraving classes contributed greatly to the development of his inclination toward graphic art.

1919 - 1922. He studied at the School of Architecture and Decorative Arts in Haarlem. The strong personality of his teacher of free graphic techniques also had a great influence on his later development as a graphic artist.

1922. He travels to northern Italy and Spain where he visits the Alhambra; in November of the same year, he publishes the booklet, Flor de Pascua, with his wood engravings.

1923. At the age of twenty-five he had his first solo exhibition in Siena and the following year he exhibited for the first time in Holland.

1924. Travels to Italy and settles in Rome.

1932. The book XXIV Emblemata with engravings by Escher is published. During ten years in Rome, he carries out numerous studies and travels: to Abruzzo, the Amalfi Coast, Calabria, Sicily, Corsica, and Spain.

1935. Leaves Italy, staying two years in Switzerland and five years in Brussels.

1941. Moved with his wife and three children to Baarn, Holland.

1951. The Studio, Times, and Life magazines publish articles about Escher.

1954. Escher exhibits at the Stedelijk Museum, Amsterdam, on the occasion of the International Mathematics Conference. That same year he exhibits at the White Gallery in Washington.

1957. The De Roos Foundation, a private printing house of limited editions, commissions Escher to write an essay on his work about the regular division in the plane. A year later the essay is published as a book with the title Regelmatige vlakverdeling (The regular division in the plane) illustrated with engravings that Escher made especially for this publication.

1959. The book Grafiek en tekeningen M.C. Escher (The graphic work of M.C. Escher.) is published.

1968. Escher makes his last graphic work: an engraving.

1972. Escher dies in Baarn, Holland, on March 27, at the age of 73.

The graphic work of Maurits Cornelis Escher

Escher's graphic production can be divided into two periods: before 1937, where landscapes predominated, and after that year, a stage characterized by a marked mathematical tendency. During this last stage, the artist developed all his creativity through themes such as regular division in the plane, perspective, regular solids and spirals, the impossible, and infinity.

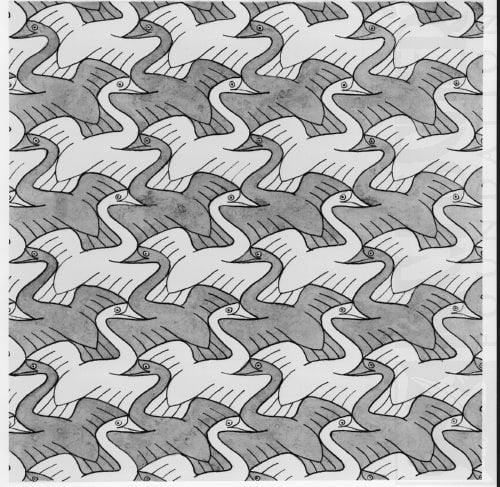
Regular division in the horizontal plane

Escher repeatedly emphasized in his writings that the regular division in the plane interested him more than anything else.

This theme has been (and still is) the richest source of inspiration for me. The symmetrical drawings reproduced here show how the surface can be divided into equal figures. The figures must limit each other, with no empty spaces.

Perspective

Escher studied perspective and its laws for many years; although he had great difficulties in representing it, he used it in a very particular way.

...already in the Renaissance it was known that not only the parallel lines of a building cut at a point on the horizon (the famous vanishing point), but also that the vertical lines converge at another point, above at the zenith and below at the nadir... but it is not until the invention of photography that we have really become familiar with vertical perspective. If we direct the lens downwards or upwards, we will recognize that it is nothing but pure convenience, on the part of those who draw works of architecture, to reproduce everything that is vertical by means of perpendicular or parallel lines. In the following engraving, the same vanishing point has several functions at the same time. Sometimes it is located at the horizon, at the nadir and at the zenith.

Impossible architectures

To exemplify this theme, the following description by the artist of one of his lithographs suffices.

At the bottom left, in the foreground, we see a sheet of paper on which a cube is drawn. Two circles mark the places where the lines intersect. Which of these lines is in front? Which is behind? To be at the same time in front and behind is an impossible thing in three-dimensional space; that is why it cannot be presented pictorially. It is possible, however, to draw an object that when seen from above offers another reality than when seen from below. The boy sitting on the bench holds in his hands a cube that exemplifies such an absurdity. He reflexively contemplates the impossible object. On the floor of the lower floor, inside the house, there is a staircase up which two people are climbing. But when they reach the top, they find themselves in the open air and have to go back into the building. Is it not surprising that neither of these people is interested in the fate of the prisoner in the cellar who is pitifully sticking his head through the bars?

Regular solids and spirals

The fact of representing three-dimensional objects in a two-dimensional plane, considering that the only reality is the three-dimensional space in which we exist, is another of the themes that most fascinated the artist. The following woodcut illustrates three closed knots made from a single ribbon whose ends have been joined together. These knots have volume, or at least give the illusion that they have a place in space, which makes it possible to observe how the ribbon is tangled to form them.

Infinite space

His need for expression led him to experiment with figures based on mathematical principles. Thanks to the study and analysis of these Maurits Cornelis Escher was able to express infinity on a surface limited by the plane.

Source: Correo del Maestro. No. 26, by Rosa Elena González