Projections from within: the mathematics behind medical imaging
When the X-ray source completes a rotation, the computer, using algorithms based on mathematical techniques, constructs a 2D image slice of the patient. This is what happens.

A simple game that amused us as children was to form figures with the shadows of our hands placed in front of a beam of light. Depending on the gestures, silhouettes of different animals would appear, or so it seemed to us. The skill consisted in knowing how to place the hands so that a certain shape, imagined beforehand, would appear.
Let us now think of a similar situation, but where it is not our hands that cast the shadows, but an unknown object. The only thing we see is the shape of the projected shadow. Will we be able to discover what the object is, or at least what its shape is?
For example, if the shadow has a circular shape, a possible answer immediately comes to mind: a ball, perhaps a soccer ball. But, after a few moments of reflection, we become convinced that it is not necessarily a ball or some other spherical object. It could also be, for example, a tin can oriented in the direction of the light, or a cooking plate. It is impossible to determine which object it is without more information.
One way to get more information is to look at the shadows in different directions. If the object rotates, we can observe how the shadow cast changes, which could help us determine the shape of the object. We could differentiate, for example, between the three possible objects mentioned. The ball will always cast a circular shadow, regardless of how it spins. If the plate rotates around one of its diameters, its shadow will become thinner until it resembles a straight line segment.
The can will cast shadows in different ways depending on its position: if it rotates around its axis of symmetry the shadow will not change, but it will change if it rotates in another direction until it projects a rectangle if it is in a vertical position in front of the light. We will then be able to decide which of the three shapes mentioned is the correct one. This, if we know a priori that it is one of these three options. If we do not have any information about the type of object, it will be difficult to find out what it is, even if we have all the possible projections.
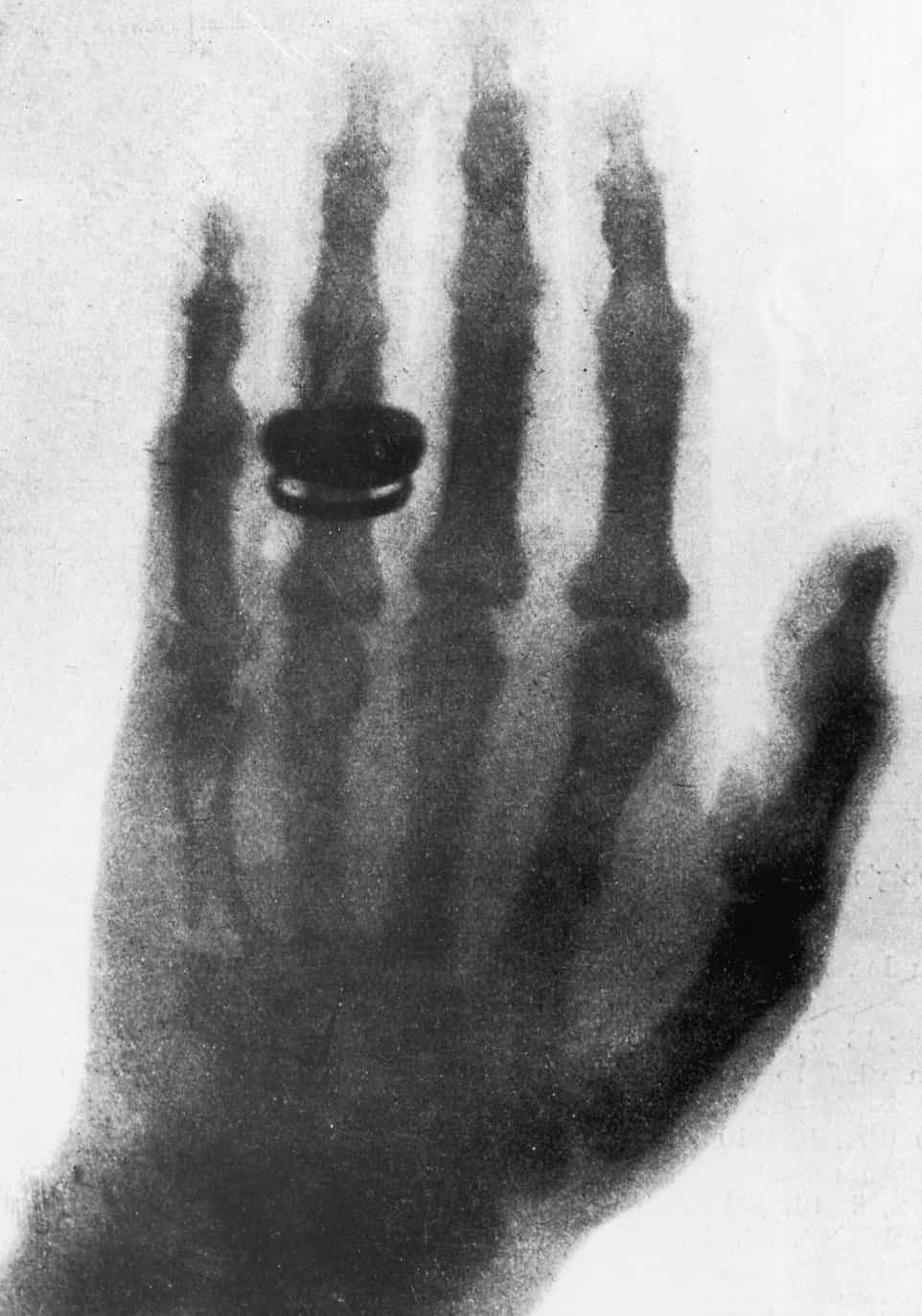
A story of many unknowns
In 1895 the German physicist Wilhelm Röntgen was working with a cathode ray tube when he noticed that a black film registered different marks. Röntgen discovered that it was caused by some kind of radiation, which passed through thin materials. He called it X-rays, being of an unknown nature, and studied its peculiarities. He found that such rays pass through soft tissue, such as skin and muscles, while they are quite attenuated by dense tissue, such as bones, and leave a mark on certain films.
This fact turned out to be of great importance since it made it possible to see our bones (or rather, their shadow on the film!), without having direct access to them. The first known X-ray was made on the hand of Anna Bertha Röntgen, Wilhelmn's wife, with a ring included. We cannot imagine Anna Bertha's surprise at seeing her own bones, the inside of her hand (she is said to have exclaimed "I am looking at my own death!"). Thanks to the discovery of X-rays, Röntgen received the first Nobel Prize in Physics, in 1901.
X-rays turned out to be the manifestation of an electromagnetic wave, like light but with a much higher frequency. During the 20th century, the use of this and other types of radiation became extremely important for the diagnosis, and even treatment, of different ailments; they are technologies in common use today. X-ray radiography had its limitations.
One of the main ones is the inability to determine the soft tissue detail of an organ. While the "shadows" of bones can be clearly seen on an X-ray, different types of tissues, such as muscle or cartilage, are hardly distinguishable. Furthermore, an X-ray is a two-dimensional image, a projection (a shadow!), of the three-dimensional object.

Remaking an object from its slices
With the idea of determining the composition of a three-dimensional object from X-ray projections, several scientists came up with the idea of creating different X-rays by changing the direction and focus of the projections. Different "slices" would be created, from which the shape of the object should be reconstructed. The basic idea of tomography (from the Greek "tomos" meaning cut or section) had emerged. It took a few years before a systematic study that would allow practical applications, in particular, because of the need for high computing power to process the various projections.
In 1966, the South African physicist Allan Cormack was working at the University of Cape Town when a fortuitous event led him to advise the Radiology Department of the city's main hospital one day a week. His assignment was to supervise the use of radiotherapy equipment, a somewhat routine activity. Cormack went further and became interested in the limitations of X-ray techniques in radiological procedures. In such procedures, the radiation was supposed to pass through homogeneous material.
But in reality, different tissues have different densities, and therefore the technique used had errors in the results obtained. Cormack thought it would be very useful to determine the density distribution inside the human body. Since tissue density is the property that determines the attenuation of X-rays, the problem of determining the density of an object from the attenuation of the rays in different directions was posed.
The problem posed by Cormack is analogous to our shadow game, only now one has not only a shadow, but the degree of attenuation of the rays, and this in each direction. It is as if we were observing not only spaces of shadow and light, but shadows in different gray scales, in all possible directions. Beyond the technical difficulty of determining the level of attenuation of the rays in all directions, he addressed the problem of determining the density of the unknown object from such information.
Cormack realized that this was a mathematical problem. He found no literature on the subject, so he set to work on solving the problem. He succeeded in mathematically determining the density of objects in a certain spatial arrangement, which was computationally implemented in several experiments. His work was published in 1963 and 1964 but did not get an immediate echo.
In the following years, Cormack learned about various works of other scientists on similar problems. Above all, he learned about the work of the Austrian mathematician Johann Radon, who already in 1917 had posed the mathematical problem studied by Cormack. Radon studied the transformation that relates a function of real values in a given region to the sum of its values along different straight lines (mathematically, it is an integral of the function on the given line).
This abstract mathematical context corresponds to a very appropriate model for the problem of lightning and its attenuations. This transformation is now known as the Radon transform. In 1917 he proved that such a transform is invertible, and determining formulas for such an inversion. This had already partially solved the problem studied (and whose solution was re-discovered) by Cormack, our gray-scale shadow problem.
Cormack also learned about the work of Godfrey Hounsfield, an English engineer who worked for EMI, Electric and Musical Industries, a company that was also dedicated to the edition of music records, an activity that years later would be its only turn and for which its acronym is perhaps known to us. The company allocated resources to build a prototype tomograph devised by Hounsfield in 1967, which was used for the first time in 1972.
As an anecdote, it is known that during those years EMI obtained great economic success from the sale of records of several musicians, particularly of a well-known English musical quartet: The Beatles. There is no certainty in this regard, but it seems that such an economic bonanza led to EMI's financial support for the construction of the first tomograph. Beyond the anecdotes, Hounsfield's merit was to design, independently from Cormack, algorithms to reconstruct an object through its projections, usable in the computers of those years.
In 1979, Cormack and Hounsfield jointly received the Nobel Prize in medicine for the invention of the computed tomography method. By that date, the technique was used in more than a thousand hospitals worldwide.
Some reflections
In this story, we find important components of scientific work. One, of course, is funding. The support of the EMI company to Hounsfield's project was decisive for the development of the first tomograph, a project that had no assurance of becoming lucrative. On the other hand, we find the importance of interdisciplinary collaboration: when Cormack was called to work at the hospital, he probably could have limited himself to supervising the work of the radiologists. However, from the needs of the physicians, he identified a scientific problem that he could help solve. Finally, we have the importance of basic research: most probably, in 1917 the mathematician Radon had no application of his research in mind. His interest lay in the mathematical significance of the problems he was studying.
However, the theory he developed has played a very important role in the improvement of the first prototype tomograph and in subsequent research on various related techniques. Cormack himself tells us, in his Nobel Prize acceptance speech, that some of the problems that he himself continued to study are, in principle, of purely theoretical interest. These studies may find an application later, but their current scientific interest justifies their existence, and in his words, that is "what science is all about".
Written by Alberto Mercado Saucedo
Dr. Alberto Mercado Saucedo is from the Department of Mathematics, Federico Santa Maria Technical University, Valparaiso, Chile.
Source: Michoacan University




